Complex mexican hat wavelet

The complex Mexican hat wavelet is a low-oscillation, complex-valued, wavelet for the continuous wavelet transform. This wavelet is formulated in terms of its Fourier transform as the Hilbert analytic function of the conventional Mexican hat wavelet:

$\hat{\Psi}(\omega)=\begin{cases} 2\sqrt{\frac{2}{3}}\pi^{-\frac{1}{4}}\omega^{2}e^{-\frac{1}{2}\omega^{2}} & \omega\geq0 \\ 0 & \omega\leq0 \end{cases}$

Temporally, this wavelet can be expressed in terms of the error function, as:

$\Psi(t)=\frac{2}{\sqrt{3}}\pi^{-\frac{1}{4}}\left(\sqrt{\pi}(1-t^{2})e^{-\frac{1}{2}t^{2}}-\left(\sqrt{2}it%2B\sqrt{\pi}\textrm{erf}\left[\frac{i}{\sqrt{2}}t\right]\left(1-t^{2}\right)e^{-\frac{1}{2}t^{2}}\right)\right)$

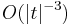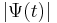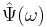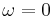This wavelet has $O(|t|^{-3})$ asymptotic temporal decay in $|\Psi(t)|$ , dominated by the discontinuity of the second derivative of $\hat{\Psi}(\omega)$ at $\omega=0$ .

This wavelet was proposed in 2002 by Addison et al. for applications requiring high temporal precision time-frequency analysis.

References

1. Paul S. Addison Wavelet Page - Low-Oscillation Complex Wavelets, P. S. Addison, et al., The Journal of Sound and Vibration, 2002